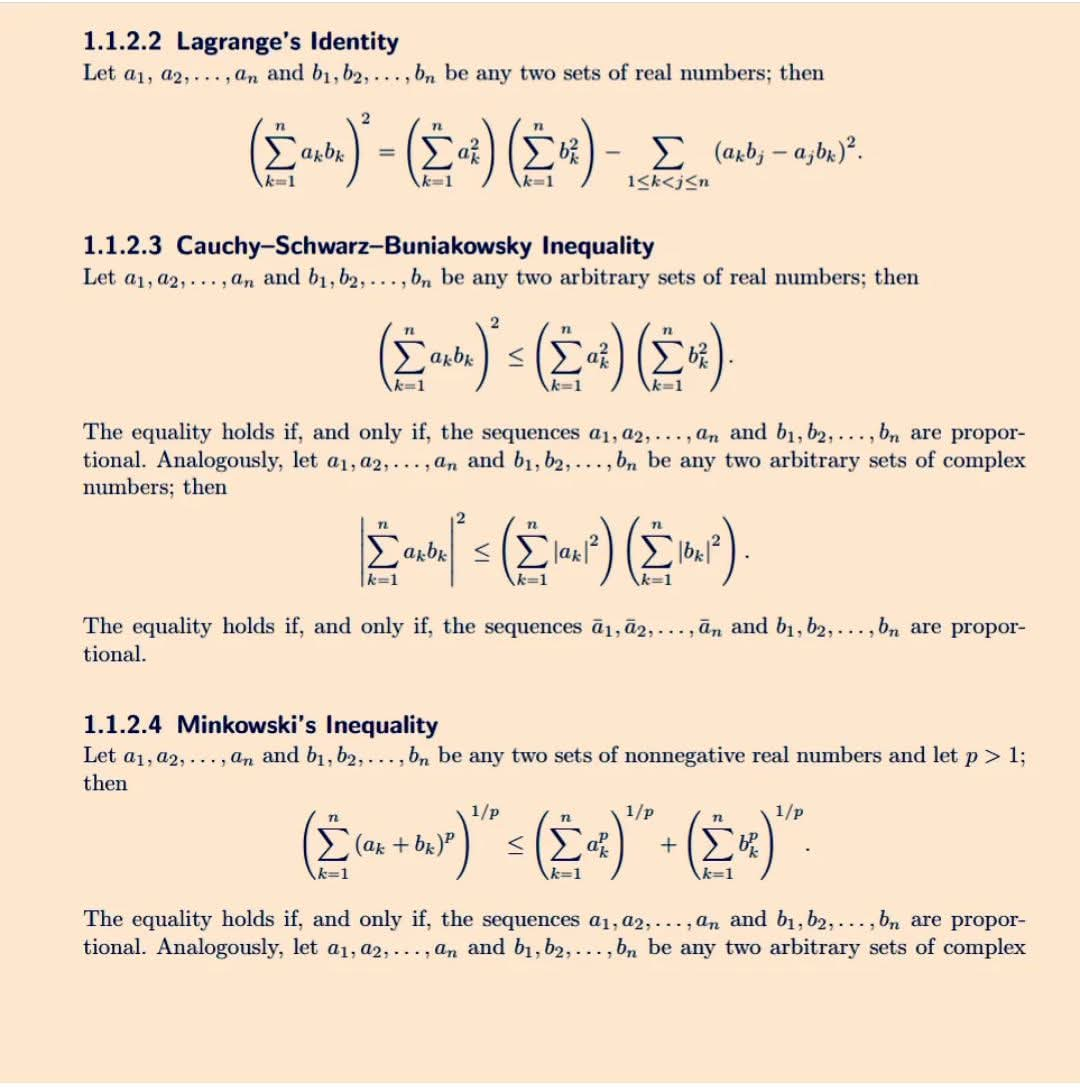
Lagrange's Identity

Lagrange's Identity

이미지에 나와 있는 세 가지 부등식에 대한 요약입니다.

1. 라그랑주 항등식:

이 항등식은 두 개의 실수 집합 (a₁, a₂, ..., aₙ)과 (b₁, b₂, ..., bₙ) 사이의 관계를 나타냅니다. 이는 각 요소의 곱의 합의 제곱이 각 집합의 제곱의 합의 곱과 같고, 교차 곱의 차이를 고려한 항을 뺀 것과 같음을 보여줍니다.

공식:

(Σᵢ₌₁ⁿ aᵢbᵢ)² = (Σᵢ₌₁ⁿ aᵢ²) (Σᵢ₌₁ⁿ bᵢ²) - Σ₁≤k<j≤n (aₖbⱼ - aⱼbₖ)²

2. 코시-슈바르츠-부냐코프스키 부등식:

이 부등식은 두 개의 수 집합 (실수 또는 복소수)의 각 요소의 곱의 합에 대한 상한을 제공합니다. 이는 곱의 합의 제곱이 각 집합의 제곱의 합의 곱 (또는 복소수의 경우 제곱 크기의 합)보다 작거나 같음을 나타냅니다. 두 수열이 비례할 때만 등호가 성립합니다.

공식 (실수):

(Σᵢ₌₁ⁿ aᵢbᵢ)² ≤ (Σᵢ₌₁ⁿ aᵢ²) (Σᵢ₌₁ⁿ bᵢ²)

공식 (복소수):

|Σᵢ₌₁ⁿ aᵢbᵢ|² ≤ (Σᵢ₌₁ⁿ |aᵢ|²) (Σᵢ₌₁ⁿ |bᵢ|²)

3. 민코프스키 부등식:

이 부등식은 두 개의 음이 아닌 실수 벡터 (수열)의 합의 p-노름을 다룹니다. 이는 기본적으로 각 요소의 합의 p제곱의 합의 p제곱근이 각 수열의 p제곱의 합의 p제곱근의 합보다 작거나 같음을 나타냅니다. 마찬가지로 두 수열이 비례할 때만 등호가 성립합니다. 이 부등식은 삼각 부등식을 일반화합니다.

공식 (음이 아닌 실수, p > 1):

(Σᵢ₌₁ⁿ (aᵢ + bᵢ)ᴾ)^(1/p) ≤ (Σᵢ₌₁ⁿ aᵢᴾ)^(1/p) + (Σᵢ₌₁ⁿ bᵢᴾ)^(1/p)

핵심 요약: 세 가지 항등식/부등식 모두 두 개의 수 집합의 요소의 합과 곱을 관련시킵니다. 코시-슈바르츠-부냐코프스키와 민코프스키 부등식은 경계를 제공하며, 등호는 수열 간의 비례라는 특정 조건에서만 성립합니다. 라그랑주 항등식은 정확한 등호를 제공합니다. 이들은 선형 대수의 기본적인 결과이며 수학 및 그 이상의 다양한 분야에서 많은 응용 분야를 가지고 있습니다.

Here's a summary of the three inequalities shown in the image:

1. Lagrange's Identity:

This identity states a relationship between two sets of real numbers (a₁, a₂, ..., aₙ) and (b₁, b₂, ..., bₙ).  It shows that the square of the sum of the products of corresponding elements is equal to the product of the sums of the squares of each set, minus a term accounting for the differences between cross-products.

Formula:

(Σᵢ₌₁ⁿ aᵢbᵢ)² = (Σᵢ₌₁ⁿ aᵢ²) (Σᵢ₌₁ⁿ bᵢ²) - Σ₁≤k<j≤n (aₖbⱼ - aⱼbₖ)²

2. Cauchy-Schwarz-Buniakowsky Inequality:

This inequality provides an upper bound for the sum of the products of corresponding elements of two sets of numbers (either real or complex).  It states that the square of the sum of the products is less than or equal to the product of the sums of the squares (or the sums of the squared magnitudes for complex numbers).  Equality holds only when the two sequences are proportional.

Formula (Real Numbers):

(Σᵢ₌₁ⁿ aᵢbᵢ)² ≤ (Σᵢ₌₁ⁿ aᵢ²) (Σᵢ₌₁ⁿ bᵢ²)

Formula (Complex Numbers):

|Σᵢ₌₁ⁿ aᵢbᵢ|² ≤ (Σᵢ₌₁ⁿ |aᵢ|²) (Σᵢ₌₁ⁿ |bᵢ|²)

3. Minkowski's Inequality:

This inequality deals with the p-norm of the sum of two vectors (sequences) of nonnegative real numbers.  It essentially states that the p-th root of the sum of the p-th powers of the sum of the corresponding elements is less than or equal to the sum of the p-th roots of the sums of the p-th powers of each sequence.  Again, equality holds only when the sequences are proportional.  This inequality generalizes the triangle inequality.

Formula (Nonnegative Real Numbers, p > 1):

(Σᵢ₌₁ⁿ (aᵢ + bᵢ)ᴾ)^(1/p) ≤ (Σᵢ₌₁ⁿ aᵢᴾ)^(1/p) + (Σᵢ₌₁ⁿ bᵢᴾ)^(1/p)

Key takeaway:  All three identities/inequalities relate the sums and products of elements from two sets of numbers.  The Cauchy-Schwarz-Buniakowsky and Minkowski inequalities provide bounds, with equality holding only under specific conditions of proportionality between the sequences.  Lagrange's identity provides an exact equality.  These are fundamental results in linear algebra and have many applications in various fields of mathematics and beyond.

#Cauchy-Schwarz-Buniakowsky Inequality
#Minkowski's Inequality
#Lagrange's Identity