왜 비행기 노선은 직선이 아니라 곡선인가요?
비행기 비행은 직선을 따르지 않고 곡선 경로를 따르며, 이 선택은 어떤 기술적인 문제를 넘어서 행성 자체의 곡률에 대한 적응이다. 기하학에서는 두 점 사이의 최단 거리가 직선이라는 것을 배우지만, 이 원리는 종이처럼 평평한 표면에만 적용됩니다. 지구의 구면을 고려할 때 두 점 사이의 최단 거리는 측지선으로 알려진 곡선이 된다.
이 개념은 곡면에 더 적합한 리만 기하학에서 유래한다. 비행 계획자들은 시간과 연료를 절약하기 위해 가장 짧은 경로를 지도에 표시하는 이 접근 방식을 사용합니다. 이 측지 경로들은 구에서 가장 효율적인 경로를 나타냅니다. 비행기는 평면도에 나타나는 것처럼 “직선”으로 비행하는 대신, 3차원 현실에서 가장 짧은 경로인 곡선 궤적을 따른다.
이 항공로들은 지구의 곡률에 대한 매혹적인 증거입니다. 모든 비행은 직관에 반하는 것처럼 보일 수 있는 경로를 따르지만, 사실 그것은 우리 구형 행성에서 가장 짧은 거리와 가장 적은 노력을 나타냅니다.
Credits: Curiosities of the Earth
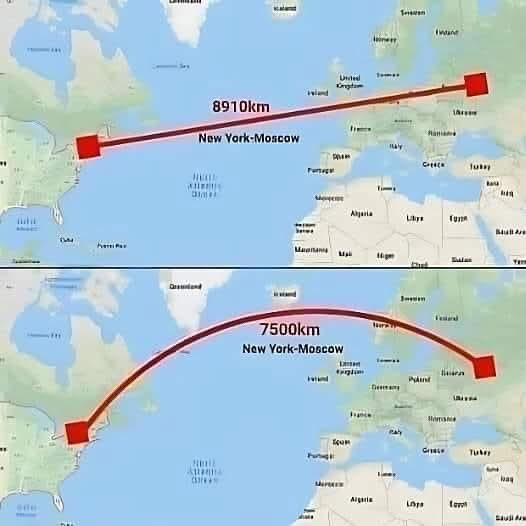
Por que as rotas de avião são curvas e não retas?
Os voos de avião não seguem uma linha reta, mas sim um caminho curvo, e essa escolha vai além de qualquer problema técnico - é uma adaptação à curvatura do próprio planeta. Em geometria, aprendemos que a menor distância entre dois pontos é uma linha reta, mas esse princípio se aplica apenas a superfícies planas, como uma folha de papel. Ao considerar a forma esférica da Terra, a menor distância entre dois pontos torna-se uma curva conhecida como geodésica.
Este conceito é originário da geometria Riemanniana, que é mais adequada para superfícies curvas. Os planejadores de voos usam essa abordagem para mapear as rotas mais curtas para economizar tempo e combustível. Esses caminhos geodésicos representam as rotas mais eficientes em uma esfera. Em vez de voar em uma "linha reta" como aparece em um mapa plano, os aviões seguem uma trajetória curva que é, na realidade tridimensional, o caminho mais curto.
Essas rotas aéreas são um testemunho fascinante da curvatura da Terra. Cada voo segue um curso que pode parecer contra-intuitivo, mas na verdade representa a menor distância e menor esforço em nosso planeta esférico.
Créditos: Curiosidades da Terra
Why are airplane routes curved and not straight?
Airplane flights do not follow a straight line but rather a curved path, and this choice goes beyond any technical issue—it is an adaptation to the curvature of the planet itself. In geometry, we learn that the shortest distance between two points is a straight line, but this principle applies only to flat surfaces, like a sheet of paper. When considering the spherical shape of the Earth, the shortest distance between two points becomes a curve known as a geodesic.
This concept originates from Riemannian geometry, which is better suited to curved surfaces. Flight planners use this approach to map out the shortest routes to save time and fuel. These geodesic paths represent the most efficient routes on a sphere. Instead of flying in a “straight line” as it appears on a flat map, airplanes follow a curved trajectory that is, in three-dimensional reality, the shortest path.
These air routes are a fascinating testament to the Earth’s curvature. Every flight follows a course that might seem counterintuitive, but in fact, represents the shortest distance and least effort on our spherical planet.
Credits: Curiosities of the Earth