벡터 미적분학의 기본 개념
아래 이미지는 벡터 미적분학의 기본 개념인 기울기(gradient), 발산(divergence), 회전(curl)에 대한 수학적 정의를 보여줍니다. 각 개념은 벡터 연산자 ∇ (del operator)를 사용하여 표현됩니다.
1. 기울기 (Gradient):
- 정의: 스칼라 함수 φ의 기울기는 벡터장으로, 각 점에서 함수가 가장 빠르게 증가하는 방향을 가리키는 벡터입니다. ∇φ로 표현됩니다.
- 수식: ∇φ = (∂φ/∂x)i + (∂φ/∂y)j + (∂φ/∂z)k
- 설명: ∂φ/∂x, ∂φ/∂y, ∂φ/∂z는 각각 x, y, z 방향으로의 φ의 편미분을 나타냅니다. 결과 벡터의 각 성분은 해당 방향에서의 변화율을 나타냅니다.
2. 발산 (Divergence):
- 정의: 벡터장 A의 발산은 각 점에서 벡터장의 소스(source) 또는 싱크(sink)의 세기를 나타내는 스칼라 값입니다. ∇·A로 표현됩니다.
- 수식: ∇·A = ∂A₁/∂x + ∂A₂/∂y + ∂A₃/∂z
- 설명: A = A₁i + A₂j + A₃k 이며, ∂A₁/∂x, ∂A₂/∂y, ∂A₃/∂z는 각 성분의 편미분입니다. 양수 값은 소스를, 음수 값은 싱크를 나타냅니다.
3. 회전 (Curl):
- 정의: 벡터장 A의 회전은 각 점에서 벡터장의 회전의 세기와 방향을 나타내는 벡터입니다. ∇×A로 표현됩니다.
- 수식: 이미지에 표시된 행렬식을 통해 계산됩니다.
- 설명: 회전 벡터의 방향은 오른손 법칙을 따르며, 크기는 회전의 세기를 나타냅니다.
요약:
이미지는 벡터 미적분학의 세 가지 중요한 개념을 간결하게 정의하고 있습니다. 각 개념은 물리학, 공학, 컴퓨터 그래픽스 등 다양한 분야에서 광범위하게 사용됩니다. 이미지의 수식은 각 개념의 계산 방법을 명확하게 보여줍니다. 더 깊이 있는 이해를 위해서는 벡터 미적분학 교재를 참고하는 것이 좋습니다.
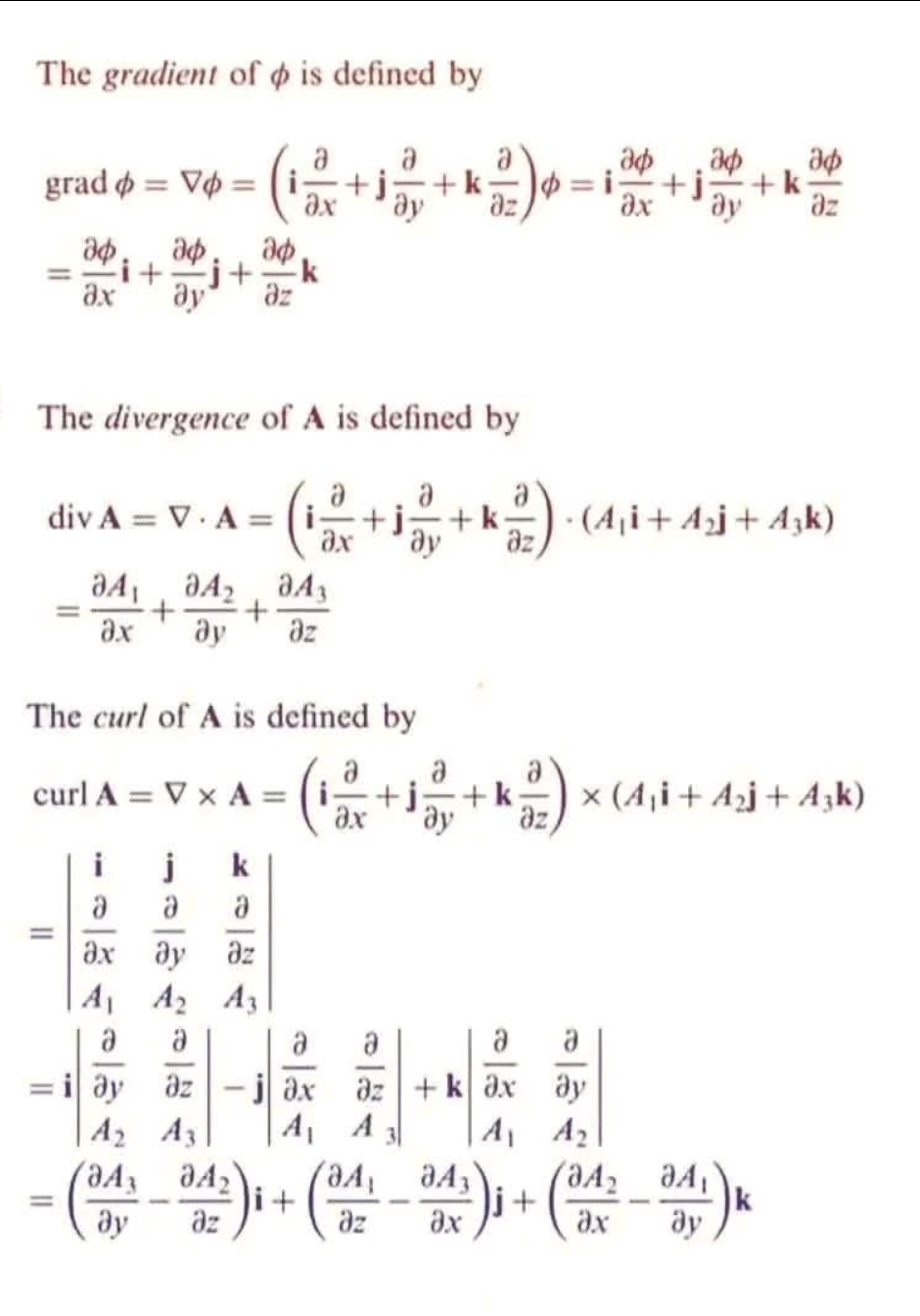
The image shows the mathematical definitions of three fundamental concepts in vector calculus: gradient, divergence, and curl. Each concept is expressed using the vector operator ∇ (del operator).
1. Gradient:
- Definition: The gradient of a scalar function φ is a vector field that points in the direction of the greatest rate of increase of the function at each point. It is denoted by ∇φ.
- Formula: ∇φ = (∂φ/∂x)i + (∂φ/∂y)j + (∂φ/∂z)k
- Explanation: ∂φ/∂x, ∂φ/∂y, ∂φ/∂z represent the partial derivatives of φ with respect to x, y, and z, respectively. Each component of the resulting vector represents the rate of change in that direction.
2. Divergence:
- Definition: The divergence of a vector field A is a scalar value that represents the strength of the source or sink of the vector field at each point. It is denoted by ∇·A.
- Formula: ∇·A = ∂A₁/∂x + ∂A₂/∂y + ∂A₃/∂z
- Explanation: A = A₁i + A₂j + A₃k, and ∂A₁/∂x, ∂A₂/∂y, ∂A₃/∂z are the partial derivatives of each component. A positive value indicates a source, while a negative value indicates a sink.
3. Curl:
- Definition: The curl of a vector field A is a vector that represents the strength and direction of the rotation of the vector field at each point. It is denoted by ∇×A.
- Formula: Calculated through the determinant shown in the image.
- Explanation: The direction of the curl vector follows the right-hand rule, and its magnitude represents the strength of the rotation.
Summary:
The image concisely defines three important concepts in vector calculus. Each concept is widely used in various fields such as physics, engineering, and computer graphics. The formulas in the image clearly show how to calculate each concept. For a deeper understanding, it is recommended to refer to a vector calculus textbook.
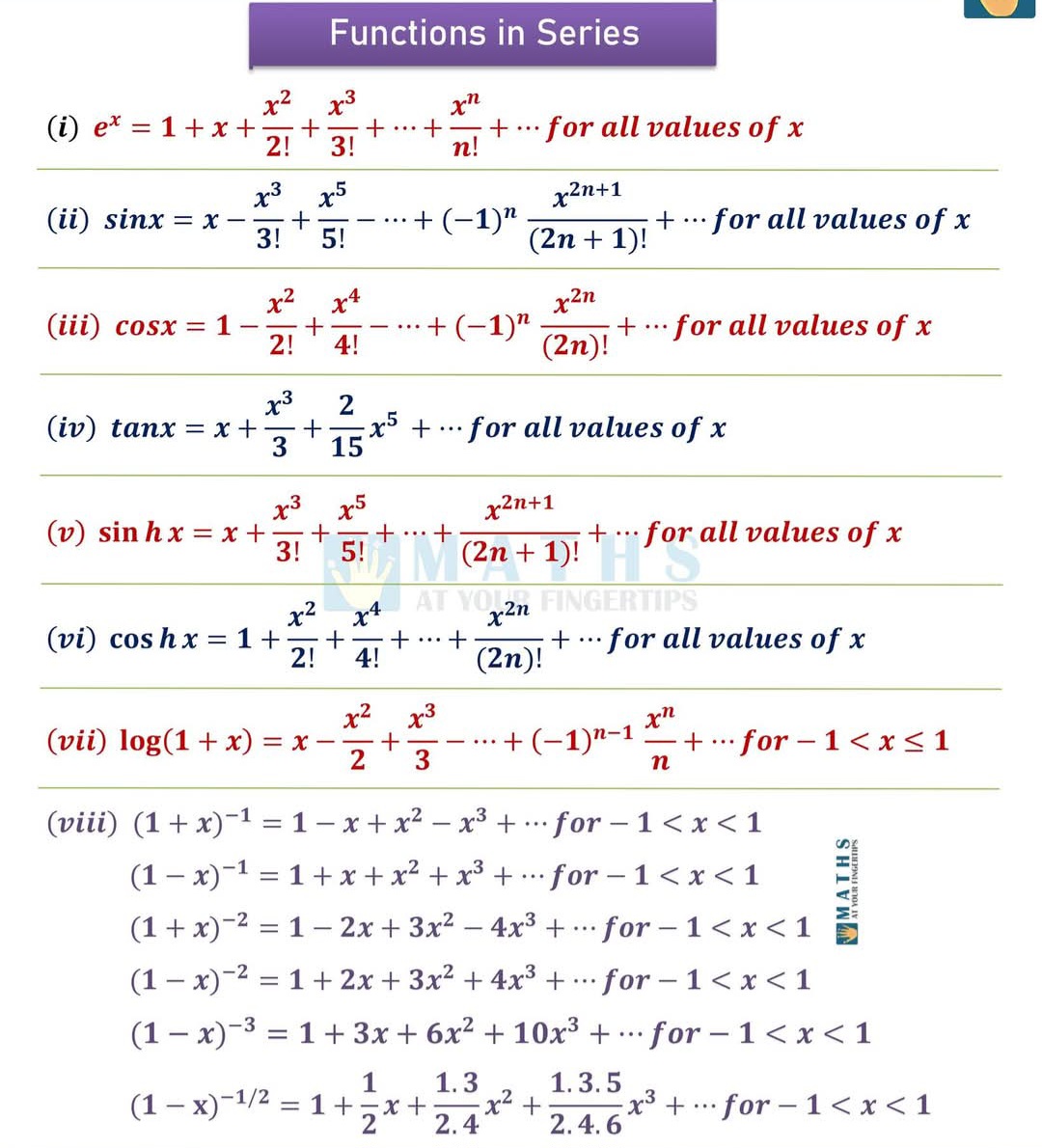
미적분학 실력향상을 위한 암기사항